Point vortex model of the snow cornice flow. The flowfield is generated by conformal mapping. The conformal map suggested by Ringleb is used. By varying the map parameter δ different geometries are generated and the existence of vortex equilibria is investigated.

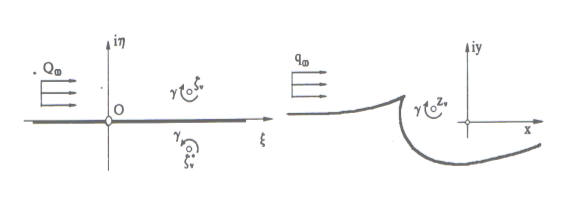
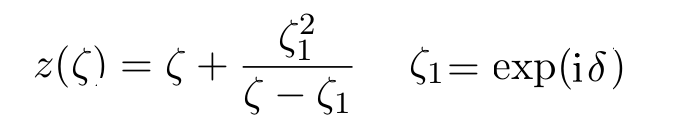
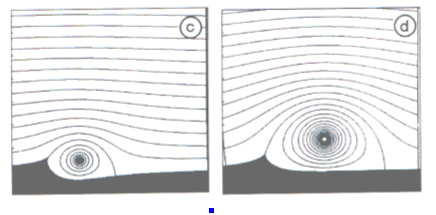
FIGURE 1: Standing vortex in a Ringleb snow cornice. Mapping planes.

FIGURE 2: Standing vortex in a Ringleb snow cornice. streamlines for (a) δ=10^o, (a) δ=30^o, (a) δ=50^o, (a) δ=70^o .

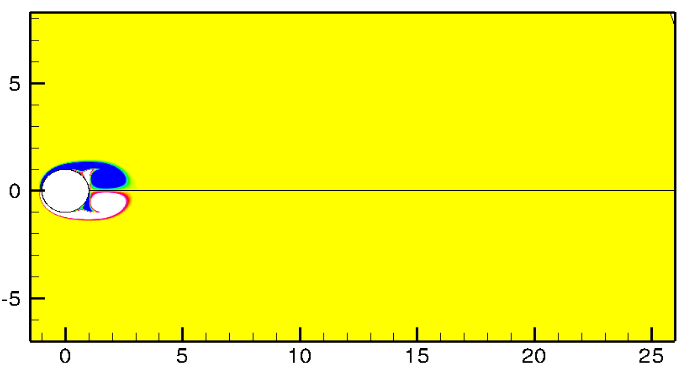
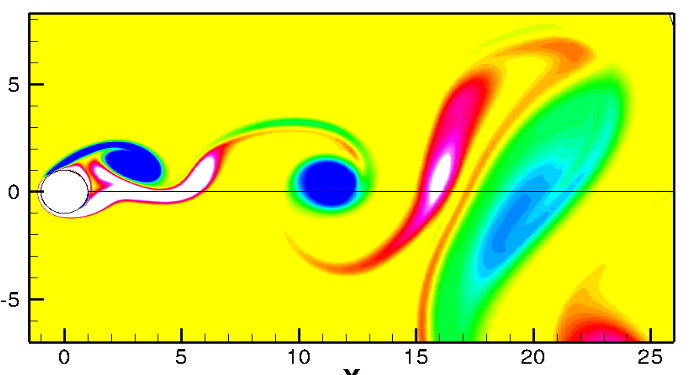
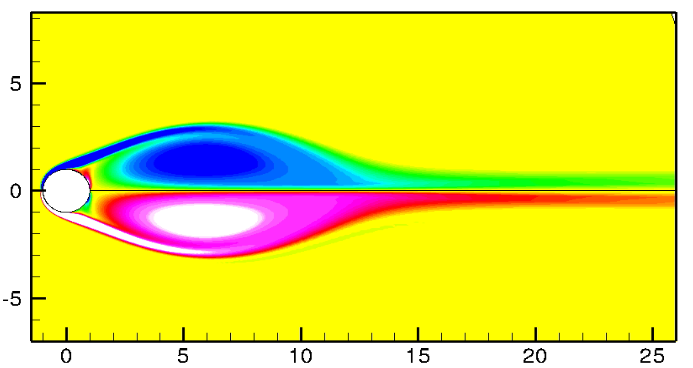
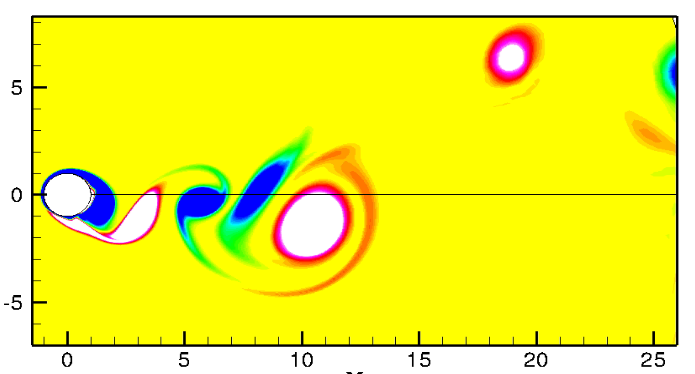
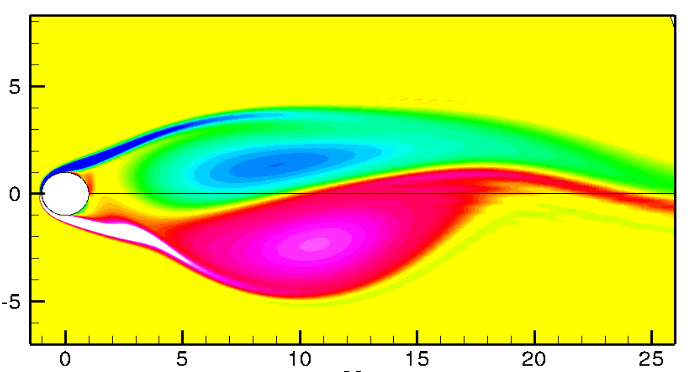
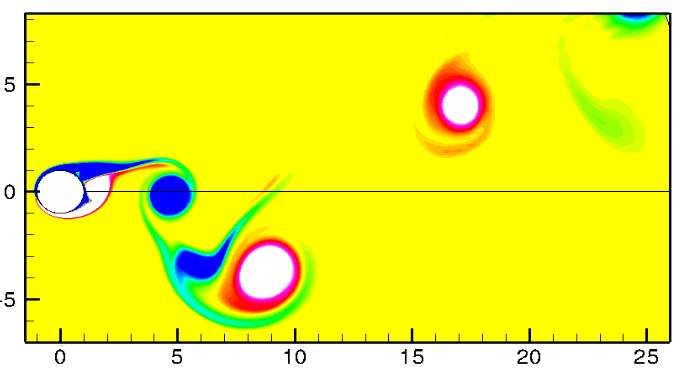
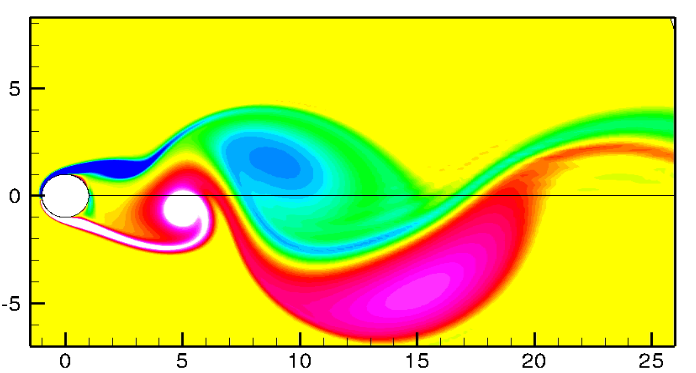
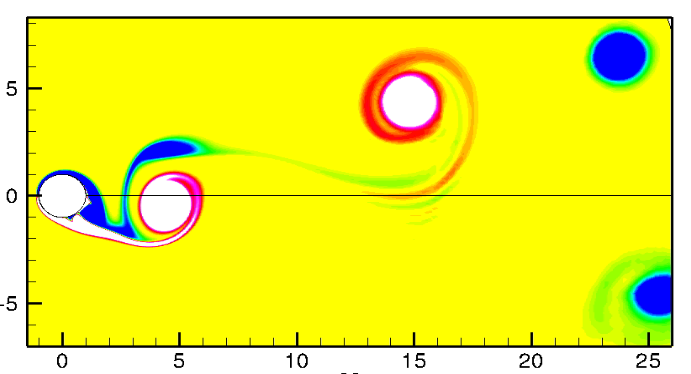
The impulsive start of a circular cylinder in a viscous fluid is simulated here by the classical vorticity-streamfunction (ω-Ψ) formulation of the Navier-Stokes equations. The generation of the symmetric vortices, the symmetry breaking, and the wake evolution are shown.

FIGURE 4: Time sequence of the impulsive start of a circular cylinder in a viscous fluid at Re = 500. Snapshots of the vorticity field.

Vortex Method is a lagrangian approach to the numerical solution of the Euler or Navier-Stokes equations expressed in terms of the vorticity equation.

Vortex Dipole Rebound

A Vortex-In-Cell formulation is used here to simulate the dynamics of a vortex dipole impinging to the wall. Results are compared with the numerical simulation in:
P. Orlandi, "Vortex dipole rebound from a wall" Phys. Fluids A, 2(8):1429-36, 1990.
based on a finite difference discretization of the Navier-Stokes equations.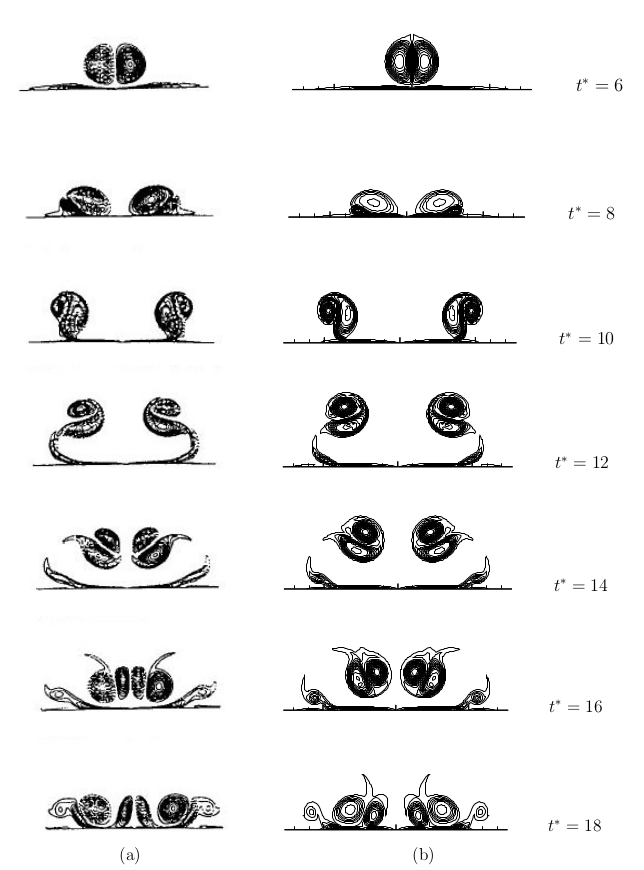

FIGURE 5: Time sequence of the impingement of a Lamb dipole normal to the wall (Re = 800). (a) Results obtained in [Orlandi], (b) present computation by using a VIC method.

Trapped Vortex Configurations

The generation of a trapped vortex in cavity flow is investigated by a Blob Vortex Method. This method is grid-free and fully Lagrangian. Compact region of vorticity in the flowfield are discretized into vortex particles (Vortex blobs) whose trajectories is integrated numerically in time. The numerical approach combines the Fast Multipole Method, to speed up calculations, and conformal mapping techniques, to simulate wall bounded flows of general shape.

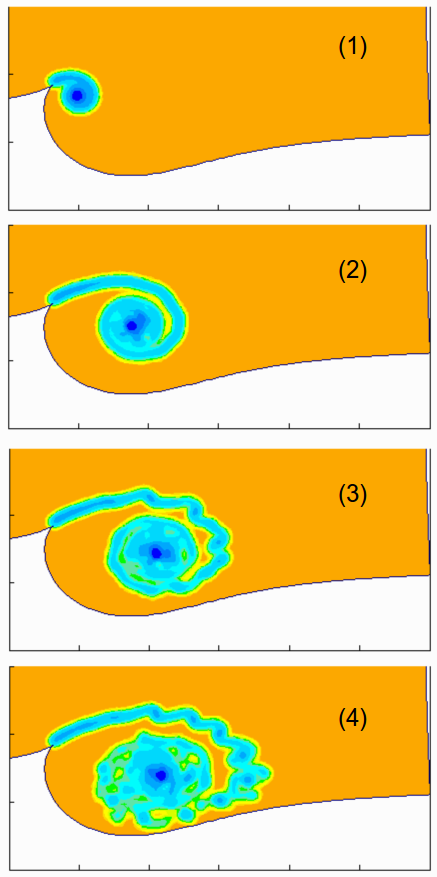
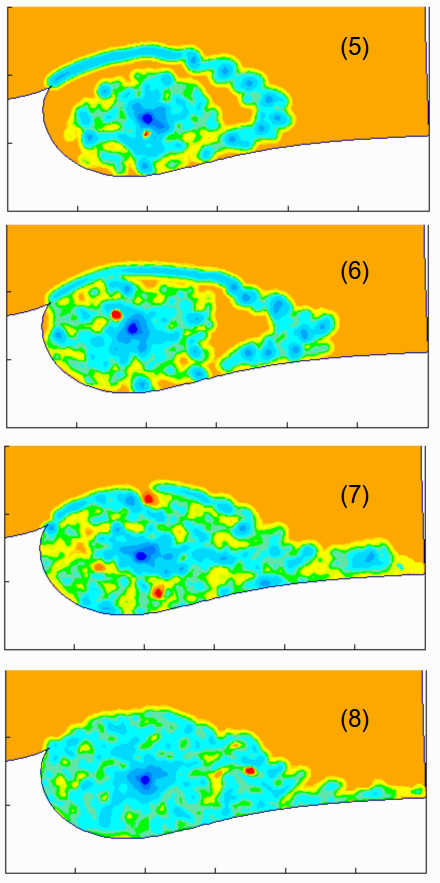
FIGURE 6: Vortex shedding from a snow cornice (Ringleb mapping with δ = 40^o ).

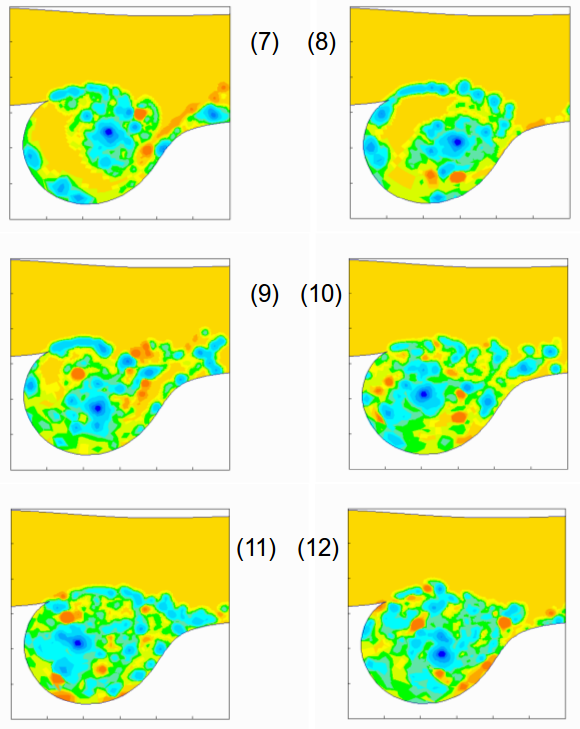
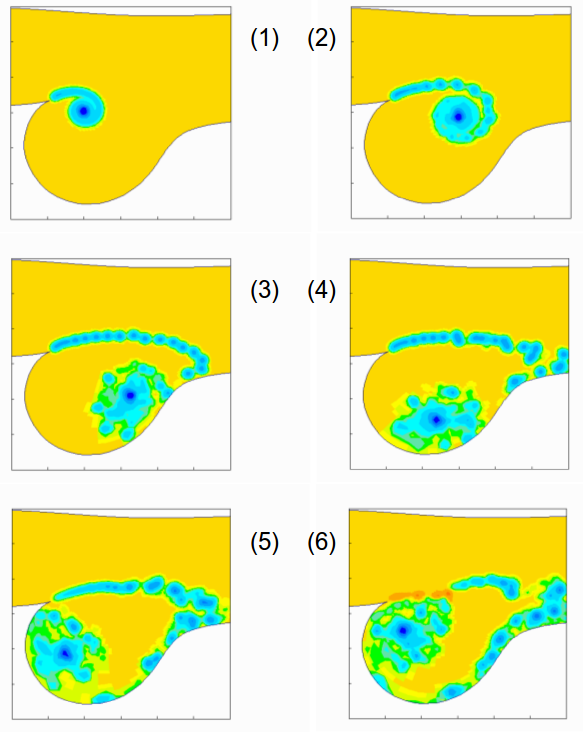
FIGURE 7: Vortex shedding from a snow cornice (Ringleb mapping with δ = 20^o ).